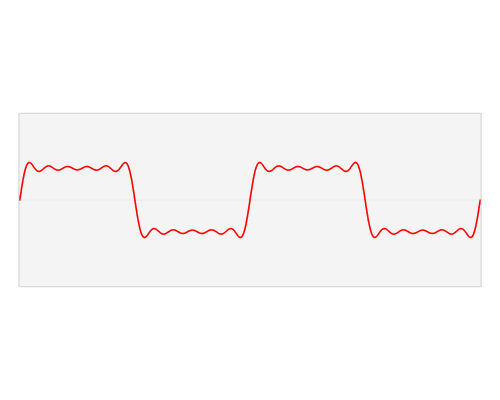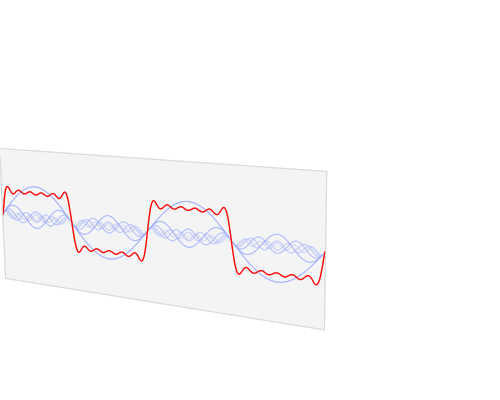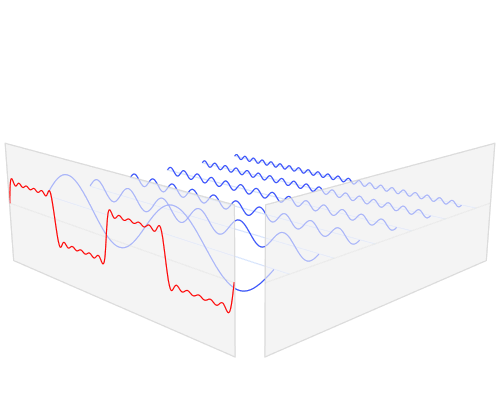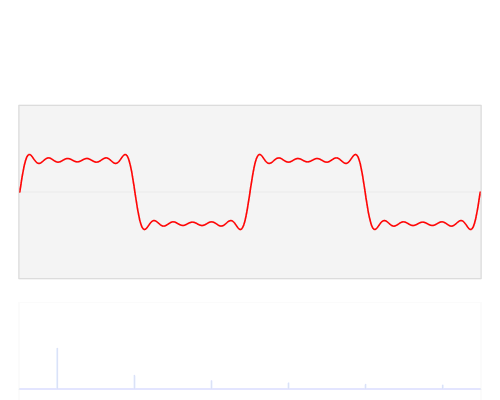
The Fourier transform takes an input function f (in red) in the “time domain” and conver

The Fourier transform takes an input function f (in red) in the “time domain” and converts it into a new function f-hat (in blue) in the “frequency domain”.In other words, the original function can be thought of as being “amplitude given time”, and the Fourier transform of the function is “amplitude given frequency”.Shown here, a simple 6-component approximation of the square wave is decomposed (exactly, for simplicity) into 6 sine waves. These component frequencies show as very sharp peaks in the frequency domain of the function, shown as the blue graph. In practice, these peaks are never that sharp. That would require infinite precision.I’m not too happy with this one yet. It’s a bit of a mixture of Fourier series and Fourier transform. The animation could also be a bit smoother in some steps. I may tweak it later. -- source link

More pics about